Interestingly, malfunction of cortisol signaling has been very strongly linked to depression. High-dose corticosteroids can produce an array of mood effects, including depression and mania, as well as other psychiatric symptoms. Relatively recently, psychoactive steroids have been approved for postpartum depression.

I tried another that I currently can’t remember the name of, and Git Ahead seemed simpler. I like that the interface is not very complex but you still get an idea what’s going on in your repo and performing tasks is intuitive.

I like Git Ahead myself.

They count it as Linux, yes!

Once the results come out, so let’s hope for the best!

No, tbh I don’t. I did okay in the written test, but I’m sure I can’t manage a conversation.

I did the reading comprehension and listening parts quite nicely, and predictably struggled with the essay. Quite likely passed though.

Surprisingly, it did go pretty well! As expected, not very great on the writing skills side, but overall OK aside from that.

Most Chinese capital is still socialized. Some privatization did indeed happen (which helped China attract foreign capital and maintain diplomatic relations), but that trend has since reversed.

Nowadays there are a few medical trends that I feel are relevant to this post. Firstly, schizophrenia is less commonly referred to as an illness, and more using the more neutral term “disorder” or even straight up “syndrome”, since an illness must have a single mechanism (known or unknown) explaining it, and we have no proof that all or even most of the cases of schizophrenia correspond to a single entity; might even say the opposite. Also, the different subtypes of schizophrenia are falling out of use, now it’s becoming more common to treat schizophrenia as a multidimensional spectrum spanning various degrees of paranoia, hallucinations, mood disorder and cognitive disorder.

I wanted to delete all the subfolders in the current directory:

 rm -rf ./*

After a few seconds, I realize in horror that I had mistyped the path. Whole system nuked. Had backups though.

“Escanciar” in Spanish means pouring from a height for the purpose of mixing a beverage (usually cider) with air. I suppose it would still be valid if you’re pouring a mix from some height.

Disabling the USB port is neat, BUT… What if it got instead reconfigured to pretend to be a USB keyboard when connected and then… you know… For research purposes, of course.

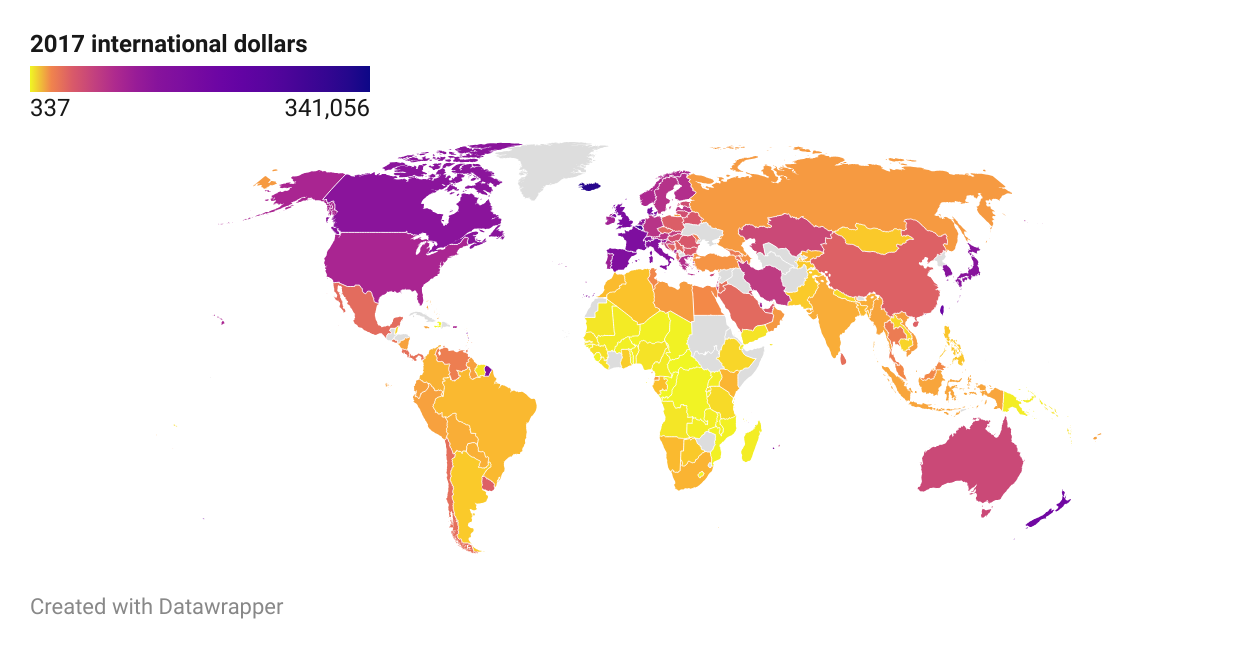
Indeed! If one person ends up spending most of their income on basic needs, they’ll not be able to spend much of it on long-lasting properties, which is what this map measures. It’s a pity though that Cuba and the DPRK did not appear in the sources, but at least we do get some interesting perspectives on the countries that do (look at Venezuela!).

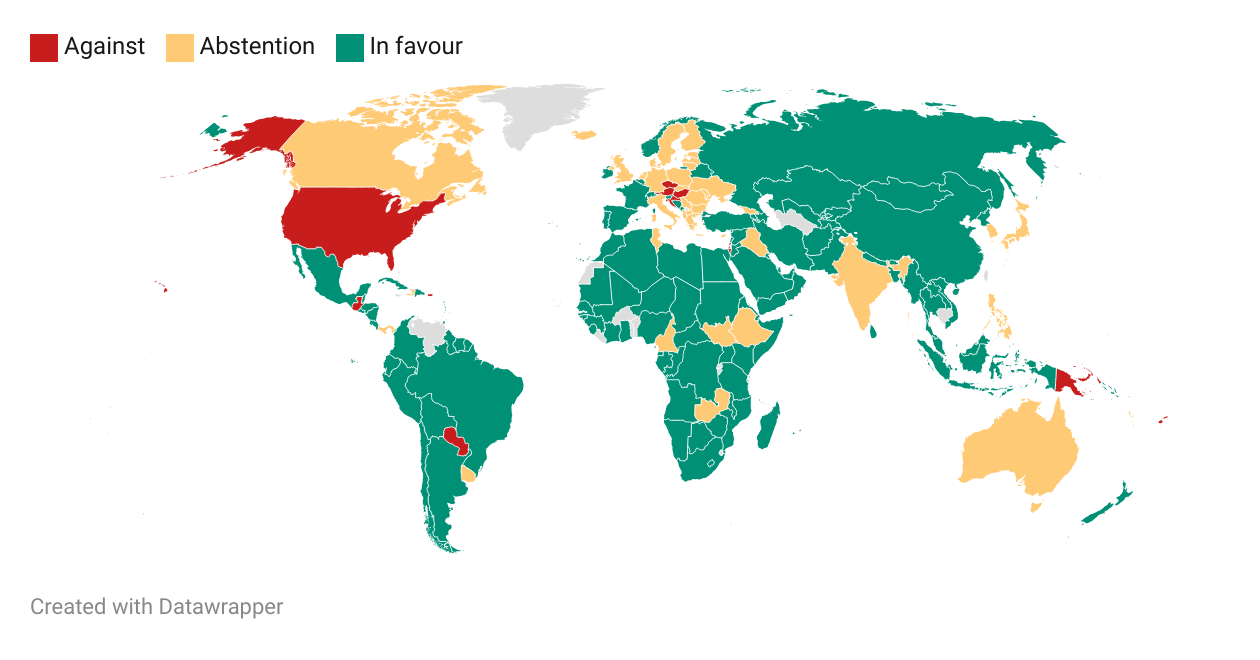
“Socialism turns out to work pretty well and is beating our ass” --> “Now, no one — certainly not me — is discounting the power of markets,” Sullivan noted at the time. “But in the name of oversimplified market efficiency, a large non-market economy had been integrated into the international economic order in a way that posed considerable challenges.”

Makes sense, thanks!

There are some really cool electoral methods that are used in some niche situations (e.g. the Schulze method). Obviously wouldn’t work to elect a government, since that would not massively benefit the ruling class as the current methods do.

Well, it’s doing it right now, so your point stands ;)

I think the methodology is not consistent before 1990, so it’s difficult to say how much of that drop is real (should probably not have included anything pre-1990).

Well, the threshold for significance might not be that strict here since the risk of seeing a difference where there is none is not really a problem. But there are very few socialist countries anyway, so our ability to tell apart real differences from coincidences is very low as you point out…